How long is infinity? Can you tell why the space is infinite? What is the division of 10 by 0? What is 10100 times of infinity? At the end of this article, you will be able to answer all these questions.
“To infinity and beyond!”

This is a very famous catchphrase. But have you ever dug deep into this? Probably not. Is it possible to go beyond infinity? Well, that is a topic for another article. Today, let us learn what do we mean by infinite. How long is infinity?
Infinity: An Amazingly Weird but Simple Concept
The concept of Infinity is very weird. It keeps the human brain wondering.

The observable range of the universe might be infinite. But can it just go on forever? It can be enormous, but it has limits. Telescopes have gone through three generations of COBE, WMAP, and Planck. Human beings tend to know the shape of the universe. Is the universe curved like a ball or flat like a piece of paper? Now, considering the universe as a curve. We can easily determine its size. But, surprisingly all three telescopes say the universe is flat. This leaves us with the concept,
Infinity is not
- Big
- Massive
- Tremendously vast
- Extremely enormous
- Infinity has no end
- It does not grow
- Infinity is not a real number
- It is Simple
What is 10 divided by 0?
Divide a number by a smaller number, it will give you bigger answers.
Example:
10 ÷ 2 = 5
10 ÷ 1 = 10
Again, ten divided by one-millionth is 10 million,
As a result, we can say, if you divide by numbers that keep decreasing up to zero, the answer will grow. It will grow up to be the largest thing possible. Then, isn’t the answer to 10 divided by zero infinity? This sounds logical.

But all we know is that if we divide 10 by a number that tends towards zero, the answer tends towards infinity. Moreover, that’s not the same thing as saying that 10 divided by zero is equal to infinity. Why not?
What do you mean by division?
Let’s dive right into the topic, shall we?
Division of Ten by two means,
“How many times must we add two together to make 10,”
or, “two times what equals 10?”
Dividing by a number is essentially the reverse of multiplying by it,
For example,
3 × 2 = 6
Again,
6 × 1/2 = 3
So the multiplicative inverse of two is one-half.
Fun Fact:
The product of any number and its multiplicative inverse is always one.
2 × 1/2 = 1
Now, if we want to divide something by zero, we need to find its multiplicative inverse. It should be one over zero.
Now,
0 × 1/0 = 0
But, in this case, the answer is zero. So, such a number is impossible. As a result, zero has no multiplicative inverse. In the end, the division of 10 by 0 is simply infinity. It is endless.
This is infinity. Simply put,
“The limit that man has not yet reached is infinite.”
What is 10100 times infinity? How long is infinity?
Infinity
When I say infinity, does any number pop up in your brain?

If so, then what are they?
Is it 1 million, 1 billion, 1 trillion, 1 quadrillion, googol, or googolplex?
Googol has 10^100 sets of numbers.
Whereas googolplex has 10^10^1000 i.e. 10^googol
As for infinity, it is far beyond that.
Again,
something × infinity = infinity
So,
10100 × infinity = infinity
These are all huge numbers. But is this infinity?
No. Absolutely not. Infinity is a cardinal number. Cardinal numbers are counting numbers. They determine how many of something. So, infinity represents the size of the set of all the numbers.
What is a number line? Is it endless?
The number line extends from negative integer to positive integer. It can extend up to “n+1” number of times. Suppose, 50 is the end of a number line. But, add 1 to it then there is more. It extends endlessly.
Then what about infinity? Is infinity also endless?
This endless set of numbers of a number line are what we know as countable infinite numbers.
Countable Infinity and Absolute Infinity
1. Countable Infinity:
It is an endless set of numbers. But, we can assign numbers to each one. So, it might extend up to infinity but it is still countable.
Infinity does not indicate any number. It is just a quantity.
2.Absolute Infinity:

First, understand this,
Are the numbers between 1 and 2 infinitely many?
Yes, this is very easy to understand.
What is the division of 1 by 3?
The answer is .33333333……… and so on.
Again,
What is the value of √2?
The answer is 1.4142135…… and so on.
Both of these are irrational numbers. They extend up to infinity. So, the numbers between 1 and 2 have infinite sets of solutions. Again, those numbers have infinite sets of solutions. As a result, we get absolute infinity.
In the end, absolute infinity is an infinite tower of infinity.
Feeling interesting? Great! So cool your head now. Because now we’ll enter the deep realm of thought.
Hilbert’s Infinite Hotel Paradox:
What is an Infinite Hotel?
Infinite Hotel is a hotel with an infinite number of rooms.
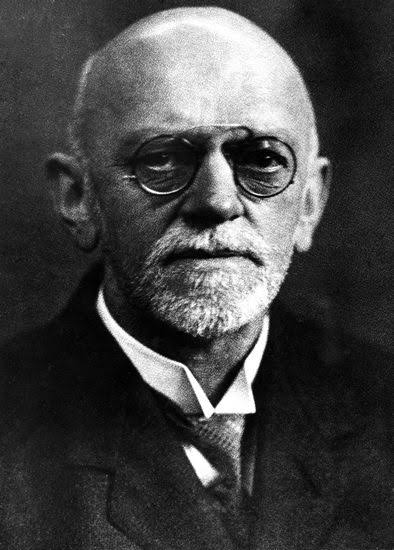
David Hilbert was a German Mathematician. Using infinity, David Hilbert created a paradox. We can say it in other words like Grand Hotel Paradox or the Infinite Hotel Paradox.
Let us imagine and learn!
Let’s dive right in.
The night manager of this hotel is ‘Jeffrey’. He is again a very good mathematician!
The hotel is full to its brim.
1. Situation 1
A new guest arrives:
At twelve-five at night, there was the arrival of a new guest. He asked Jeffrey for a room. But all the rooms of the hotel are full. Again, since Jeffrey is a mathematician, he found a mathematical solution.
Jeffrey then told the people in each room to move to the next room. That is, the man in the 1st house will go to the 2nd house. Again, the man from the 2nd house will go to the 3rd house.

So, the man from the nth room will go to the n + 1th house.
As a result, the first room was empty. So, the new guest got his room.
You have a question right?
Which room will the man in the last room of the hotel go to?
This is an Infinite Hotel. It is endless. Because
Infinity+1 still equals infinity.
2. Situation 2
A bus of infinite length with an infinite number of guest arrives:
The infinitely long bus perplexed Jeffrey at first but he calms himself down, saying that he was a good mathematician. Since Infinite means an enormous number of guests. They will need an infinite number of rooms. Jeffrey then said to himself,
‘I have to create an Infinity inside an infinity.‘
Multiplying any number (whether it is even or odd) by 2 always gives an even number. So if we multiply n by 2, we get 2n. As a result, Jeffrey told people in each room to go to another room. He gave them a room number twice the number of their current room number.
So, the man in room 1 will go to room 2. The man in room 2 will go to room 4. The man in room 3 will go to room 6.

In this way, the person in the nth room will go to the 2n number room.
As a result, an odd number of rooms will be empty.
We know,
The set of even numbers is infinite (2, 4, 6, 8 ……)
The set of odd numbers is also infinite (1, 3, 5, 7….).
So, Jeffrey then told the infinite new guests to go to the infinite new odd number of rooms.
3. Situation 3
An infinite line of infinitely long buses arrives:
At three o’clock in the night, an infinite line of some Infinitely long buses arrives. Again, each of them has an Infinite number of passengers. Now what?

At that moment, Jeffrey’s eyes fell on the picture of Euclid, the great mathematician on his desk.
Around 300 B.C. Euclid proved,
The set of prime numbers (numbers that can only be divided by 1 and numbers such as 2,3,5,6,11,13) is infinite.
Jeffrey said,
‘I have to create an infinite number of new infinities inside my infinity.’
So, now what did Jeffrey do?
He moves all the current guests in the hotel, to the power of the first prime number 2. That is, the guest in room 2 will be asked to go to room 22 = 4 number room. The person in room 3 will go to room 23 = 8 number room. The person in room 4 will go to room 24 = 16 number room.
So the person in room n will go to room 2n room.
Next, for the guests of the first bus,
Their rooms will be according to the power of the second prime number of the world i.e 3. In this process, the guest in seat number 1 will go to room 31 = 3 (notice that room 3 is already empty). Again, the person in seat 2 will go to room 32 = 9. Similarly,
The person from seat n will go to room 3n.
There might be a question in your mind now. Room 9 might not be empty, then what?
Fun Fact:
The power of two prime numbers is never the same.
i.e. 2x ≠ 3y.
In this way, you will be able to empty an infinite number of rooms for everyone on the first bus.
Similarly, for everyone on bus 2, they will go to the hotel room by hitting Prime number 5,
Everyone in bus 3 will go to prime number 8. Everyone in bus 4 will be told to go to 11.
How much did Jeffrey earn?
Suppose Jeffrey’s salary is one dollar per room. At the very beginning, each of the infinite houses was full. Then Jeffrey got an infinite amount of money as a salary. Then after the huge boom of an infinite number of guests, Jeffrey got another Infinite number of dollars from each person.
So,
What is the sum of his salary?
Infinity + Infinity = Infinity
So Jeffrey regrets it a little bit. He previously had infinite money. Even after so much hardship, he has that infinite amount of money. So, there has not been any increase in money at all!
How is Infinity Affecting our lives!
We make a lot of choices in life. Moreover, these choices are infinite in number.
In the words of Deepak Chopra,

“You and I are infinite choice-makers. We live in a field of all possibility wherein all moments we have access to infinite choices.”
Conclusion:
I hope you enjoyed my ultimate guide to infinity.
Did you understand everything at once? Do you want to be the manager of an infinite hotel like Jeffrey? Or, will it be like a nightmare for you? Either way, let me know by leaving a quick comment below.
I wanted to conclude this article with a joke about infinity. But, surprisingly I couldn’t come up with any ending!
Read More Exciting Articles